Chops: Intermediate
Theory: Intermediate
Lesson Overview:
• Develop a wider chord vocabulary.
• Understand the theory behind chord construction..
• Discover practical uses for unusual chords.
Click here to download a printable PDF of this lesson's notation.
As you explore new shapes and sounds on the guitar, do you ever wonder exactly how many chords are possible in standard tuning? Or even how many chords there are in the spectrum of the musical universe? First, let’s clarify. You can, of course, play only six notes at a time on a 6-string guitar. For the purposes of this lesson, we’ll only talk about chords with at least three different notes. (Sorry power chords, no offense.)
As it turns out, this question can easily be answered with a math equation. I’m horrible at math, but I have a couple of friends who are math whizzes—Nick Didkovsky (a guitarist who is also a mathematician) and John Charpie (who is a physicist). To them this equation is simple; to me not so much, but I get the basic idea. I thank them from the bottom of my heart for helping me figure some of this out.
Using the 12-note chromatic scale, here are the combinations available to us.
1 note = 12 ways (not a chord) 2 notes = 66 (not a chord) 3 notes = 220 4 notes = 495 5 notes = 792 6 notes = 924 7 notes = 792 8 notes = 495 9 notes = 220 10 notes = 66 11 notes = 12 12 notes = 1
Total = 4017 Total playable guitar chords = 2341
Now you might be asking, are these stats of any practical use? Well, perhaps not directly. But they do illustrate how many possibilities musical notes have to offer, and suggest how very little musical territory has been explored so far. Of all the music in the world, 99 percent is derived from essentially the same harmonic theory and half dozen scales. The unexplored musical possibilities these statistics illustrate comprise a topic that requires a longer conversation and more complex theoretical understanding. For now, I just wanted to help you understand that there’s more than one way to carve an octave, melodically and harmonically. And while it’s open to debate whether many of these options sound good, keep in mind that we tend to like what sounds familiar, and composers and players have experimented with only a handful of these choices.
The Chords
Fig. 1 contains 35 different three-note chords that live within the C major scale (C–D–E–F–G–A–B). Disclaimer: I think there are too many chords on this chart! It has been proven that the more options humans have, the more difficult it is to make a decision. It’s highly likely you will play through this chord chart and then never look at it again because its practical application is vague at best. I don’t want that to happen.
Play through all the chords, then pick one (only one) you like and start using it. Heck, play through the first five chords, then pick one you like and start using it. Don’t let this chart overwhelm you. While it can be fun, it can also be a lot like reading a dictionary.
Be aware that the example above contains only one voicing possibility (i.e., the order of notes) for each three-note chord. There are actually six different ways to voice each one of them. You also have the option to duplicate notes in different octaves. While this does not make our options infinite, I think you get the point without asking my math buddies to compute them. I have laid the chart out somewhat systematically; see if you can figure out how it works.
The reason I have only identified the chords C, Dm, Em, F, G, and Am is because those are the traditional triads (three-note chords formed by stacking thirds or every other note from the scale) that are not open to debate. C, E, and G will always make a C major triad. The same goes for the other triads. On the other hand, chords that are not strictly stacked thirds are open to interpretation and naming them depends on their relationship to other chords. For instance, the third chord in this chart looks like a Cmaj7 (C–E–G–B) to me, even though it has no 5th. But it could also be thought of as an Am9 (no root), Em6 (no third), and even Bsus4(b9). This last one is a bit obtuse, more theoretical, and less practical.
A Chord by Any Other Name
Okay, okay, I can hear you out there yelling, “Name the rest of those chords!” But how should we go about naming all those other triads/tri-chords? At the risk of contradicting what I said earlier, I’ve decided to go with what I consider to be the most common function of each chord (Fig. 2). Some are easier than others, the Fsus2 in measure two is obvious to me, even though we could also call it Csus4, G7sus4, and a few others without root notes! If you know your theory, you should feel free to disagree with me on some of these names, otherwise you’re just going to have to trust me.
A very common example of a chord having more than one name is the second chord of the fifth measure. Even though I called it a G7, it’s more of a B diminished because that chord contains no G note! But the other three notes imply the sound of G7. If you find this a difficult concept to grasp you are not alone, this is somewhat complex, but if you use your ear, you can tell that this G7, even without a G, sounds and functions the same as a full G7.
Fig. 3 is an example of the common G7 “cowboy chord” moving to C. Then you can hear the G7 tri-chord moving to C. Can you hear it functioning in the same way? Finally, we have the same G7 tri-chord moving to a different C triad, with smoother voice leading.
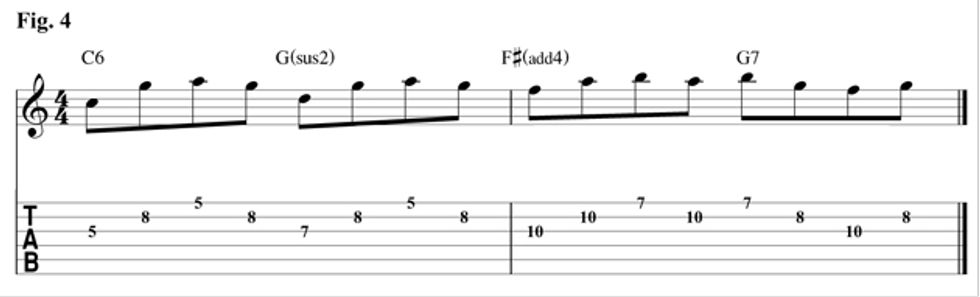
I find the easiest way to put any chord into immediate, functional use is to play it in the context of a common chord progression. Fig. 4 is your everyday I–IV–V–I in the key of C. These particular voicings and the arpeggio pattern provide a certain ’90s pop sound to the progression.
Next, in Fig. 5, is a minor Im–IVm–Vm, to which I have added open-string bass notes to emphasize the harmony. This would work nicely as an intro to a late ’80s metal tune.
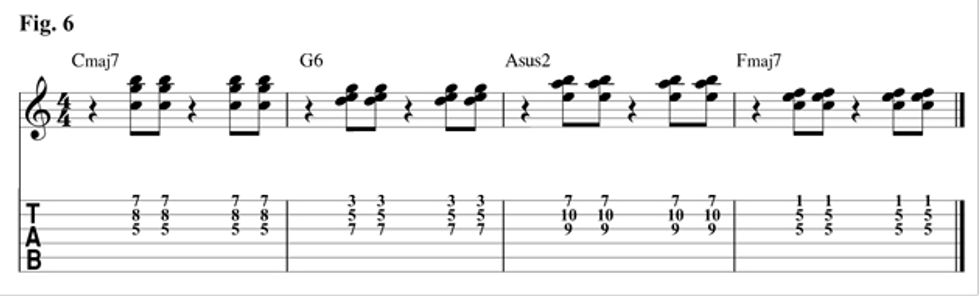
Finally, Fig. 6 demonstrates some alternative voicings for the ubiquitous I–IV–VIm–V found in so many pop songs from the last 60 years.
These common points of reference are just the beginning. You don’t have to use these everyday progressions in your own songs. Develop some new, unique sounding series of chords that will leave listeners wondering, “What chord is that?” The more harmonic ambiguity the better I say!













![Devon Eisenbarger [Katy Perry] Rig Rundown](https://www.premierguitar.com/media-library/youtube.jpg?id=61774583&width=1245&height=700&quality=70&coordinates=0%2C0%2C0%2C0)















